Trimming down Perucetus

Further trimming down the marine heavyweights: Perucetus colossus did not come close to, much less exceed, the tonnage of blue whales, and the latter are not ultra-sized either

Gregory S. Paul and Asier Larramendi

Article number: 28.1.a6
https://doi.org/10.26879/1435
Copyright Palaeontological Association, January 2025

Author biographies
Plain-language and multi-lingual abstracts
PDF version

Submission: 5 August 2024. Acceptance: 26 December 2024.

ABSTRACT

Extrapolating from skeletal/total mass ratios, the gigantic Paleogene whale Perucetus colossus has been estimated to have massed 85 to 341 tonnes, approaching and even exceeding the blue whale (Balaenoptera musculus). Such a large variation in body mass lacks the precision needed for analytical accuracy and is therefore not of technical value. Despite sufficient remains for a much more accurate volumetric model, none was produced for robust testing their procedure. A subsequent paper downscaled Perucetus to 60 to 70 tonnes as the most probable estimate. To better assess the question, we have produced multiview profile-skeletals for Perucetus and other large marine mammals in the most extensive such effort to date. These include the first accurate restorations of a number of large marine mammals. Perucetus is restored using the proportions of heavy boned pachycetine basilosaurs. Cross comparisons and attempts to illustrate the extinct whale's realistic volume leave no doubt that the Perucetus holotype did not reach the cetacean heavyweight category. A length of 15 to 16 m and a mass of 35 to 40 tonnes is more in line with its known anatomy. This result was affirmed by recalculations of skeletal/total mass relationships in large pachyosteosclerotic marine mammals, which suggest the method can produce useful estimates if conducted properly. Although their initial size expansion was remarkably rapid, basal cetaceans did not balloon to super whale dimensions just a few million years after the initial evolution of the fully marine forms. Evolution of extremely large body size exceeding 50 tonnes did not occur until the late Neogene. The biggest whale of all time, the blue, is not likely to exceed ~30 m and 200 tonnes. It is emphasized that anatomical knowledge translated into technical volumetric models remains the most critical means of restoring the mass of extinct organisms.

Gregory S. Paul. 3100 St Paul St 604, Baltimore, MD 21218, USA. GSP1954@aol.com
Asier Larramendi. Eofauna Scientific Research, Beloka 5, 2 eskubi, Donostia 20009, Basque Country, Spain. larramendi.asier@eofauna.com

Keywords: body mass estimation; basilosaurids; cetaceans; water displacement; graphic double integration; skeletal restoration

Final citation: Paul, Gregory S. and Larramendi, Asier. 2025. Further trimming down the marine heavyweights: Perucetus colossus did not come close to, much less exceed, the tonnage of blue whales, and the latter are not ultra-sized either. Palaeontologia Electronica, 28(1):a6.
https://doi.org/10.26879/1435
palaeo-electronica.org/content/2025/5431-trimming-down-perucetus

Copyright: January 2025 Palaeontological Association.
This is an open access article distributed under the terms of the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original author and source are credited.
creativecommons.org/licenses/by/4.0

INTRODUCTION

It has long been understood that the extant blue whale (Balaenoptera musculus) is the largest living animal, if not of all time. Blue whales and other gigantic whales evolved in response to the optimal feeding conditions created by the ice-age oceans during the late Neogene period (Slater et al., 2017). However, the view that the blue whale is possibly the largest animal of all time was challenged twice in 2023. Paul and Larramendi (2023) explained that fragmentary remains suggest that the sauropod dinosaurs of the Jurassic and Cretaceous rivaled blue whales at 110 to 170 tonnes. That result was derived from the gold standard of most reliably estimating masses of extinct creatures, anatomically rigorous volumetric modeling. Then, Bianucci et al. (2023) contended that at estimated masses of 180 to 340 tonnes based on living cetaceans’ skeletal fractions, the partial remains of the gigantic Paleogene stem-whale Perucetus colossus “fall in or exceed the body mass distribution of the blue whale, therefore challenging the blue whale’s title of heaviest animal that ever existed.”  This conclusion received wide coverage in the science news media. But the appearance of a super-sized whale so shortly after the first tiny cetaceans would be an evolutionarily extraordinary event. Skeptical of that possibility, Motani and Pyenson (2024) used a combination of volumetric modeling and skeletal/total mass calculations to show that Perucetus colossus could not have been as large as blue whales. Motani and Pyenson (2024) then went on to estimate that the latter reached 270 tonnes at 33 m, in contrast to the ~30 m and ~200 tonnes maximum arrived at by others for Balaenoptera musculus (McClain et al., 2015).

Bianucci et al. (2023) used novel, elaborate skeletal/total mass extrapolations to estimate the mass of the Perucetus colossus with very inconsistent results, varying from 85 to 341 tonnes. That the results cover such very large plus-minus factor should cast doubt on their procedure, at least as conducted by Bianucci et al. (2023). That the preserved vertebrae and especially ribs of P. colossus are smaller in dimensions that those of B. musculus further reduces confidence in the Bianucci et al. (2023) results.

Modern scientific methods for reconstructing masses through volumetric models based on precise skeletal restorations have been developed over recent decades to produce results avoiding outcomes from improper anatomical models or methodologies, like limb bone strength, which do not apply to marine tetrapods (Paul 1997, 2019; Larramendi, 2016; Brassey, 2017; Larramendi et al., 2021; Motani et al., 2023; Romano et al., 2023; Motani and Pyenson, 2024). When restoring the mass of a taxon known only from very incomplete remains, it is critical to as accurately as possible restore and model it and any available relations for purposes of comparison and extrapolation. In particular, extraordinarily high mass estimates must always be documented by and shown to be plausible with technical profile-skeletals. This benchmark method is used to further test the Bianucci et al. (2023) and the Motani and Pyenson (2024) methods and results. In order to do so, the most extensive effort to date to accurately restore the body forms and masses of extant giant whales based on mounted skeletons and photographs is conducted. Aside from addressing issues regarding modern cetaceans in Motani and Pyenson (2024), this is itself an issue of importance because there has yet to be a consensus on the appearance of living mega cetaceans such as the blue whale. While the results herein support one of the two cited papers much more than the other, they raise issues with both.

ABBREVIATIONS

Institutional Abbreviations

LML Long Marine Laboratory at the University of California, Santa Cruz
NHMB Natural History Museum Barcelona, Barcelona
MNHN Museum national d’Histoire naturalle, Paris
MSC Museo de las Salinas del Carmen, Carmen
MUSMED Museo di Storia Naturale del Mediterranean, Livorno
NHMW Natural History Museum Vienna, Vienna
SCF Senda de los Cetáceos de Fuerteventura, Morro Jable
SMNKU State Museum of Nature, Kharkiv National University, Kharkiv
UZMH Finnish Museum of Natural History, Helsinki
ZMUC University of Copenhagen Zoological Museum, Copenhagen

Other Abbreviations

Ca, caudal; GDI, graphic double integration; L, lumbar; ln, natural logarithm; NSG, neutral specific gravity; SG, specific gravity; SF, skeletal fraction; T, thoracic; WD, water displacement.

PLUS/MINUS ERROR RANGES MUST BE REASONABLY NARROW TO HAVE PRACTICAL VALUE

A basic, core goal of science is to narrow +/- errors to zones narrow enough to make them of practical use. For example, in chronostratigraphy, error margins can be as small as a few percent (Renne et al., 2013). Estimating the masses of organisms cannot be as precise as radiometric dating, as the mass of a given healthy adult individual can fluctuate up to 10% daily due to feeding, drinking, and defecation, and by 25% or more seasonally due to environmental and physiological factors (Paul, 1997, 2019; Lister and Stuart, 2010, 2015; Larramendi, 2016; Christiansen et al., 2018). Because the Bianucci et al. (2023) results vary fourfold for a particular individual they do not provide a mass estimate sufficiently constrained to be of practical use, and are potentially misleading. This variability is even greater than that typically seen when estimating body masses of terrestrial vertebrates from the strength of their proximal limb bones, where the +/- errors can exceed twofold. (Paul, 1997, 2019; Larramendi, 2016; Brassey, 2017; Romano et al., 2023). It also exceeds the range of estimated body masses for living cetaceans calculated via length-weight regression equations, where the real weights fall close to the regression lines and typically within the prediction intervals (Bigg and Wolman, 1975; Lockyer, 1976; Mikhalev, 2019). However, this method cannot be directly applied to extinct species. In contrast, recent volumetric modeling has an error range of about a fifth combined plus or minus in complete skeletons, quantitatively adequate for the biological purpose (Paul 1997, 2019; Larramendi, 2016; Romano et al., 2023).

BIANUCCI ET AL. (2023) PROCEDURE AND REMARKS

Bianucci et al. (2023) aimed to estimate the body mass of the Perucetus colossus type skeleton (MUSM 3248), which displayed an exceptionally wide range of potential masses, spanning from 85 to 341 tonnes. To achieve this, they employed a two-step approach. On one hand, they established a bivariate relationship between the dry skeletal mass and the body mass of various extant aquatic mammals, including whales and sirenians. On the other hand, they estimated the dry skeletal mass of P. colossus by relying on the scanned type skeleton of the late Eocene Cynthiacetus peruvianus (MNHN.F.PRU10) and adjusting its proportions to match those of P. colossus. As a result, they determined the skeletal mass of P. colossus, ranging from 5.3 to 7.6 tonnes, depending on the total vertebral number assumption. The lowest skeletal mass was derived assuming 12 thoracic and 15 lumbar vertebrae for Perucetus colossus, a reasonable possibility. However, the upper estimates assume a very high thoracic and lumbar number observed in Cynthiacetus peruvianus, which is highly improbable in pachyosteosclerotic species (see Uhen, 2001, Gingerich et al., 2022). The highest body mass estimate (341 tonnes) was derived by combining the highest skeletal mass estimate (7.6 tonnes) with the lowest SF (the ratio of skeletal dry mass to total body mass) from their database found in the extant cetacean Mesoplodon europaeus. On the contrary, for the lowest body mass estimate of P. colossus, they utilized the mean SF of 6.25%, which was derived from 11 adult extant Trichechus manatus sirenians (Bianucci et al., 2023, supplementary data 2). However, if one employs the most extreme SF (8.14%) found in T. manatus from the dataset, as they did for whales, the lower-end estimate for the body mass of P. colossus would have decreased to 65 tonnes. They also attempted to estimate a potential mean body mass of 180 tonnes for P. colossus, relying on the mean SF of 3.6% found in whales. Nevertheless, it is important to note that their procedures have several issues, which will be discussed in detail below.

Perucetus colossus Skeleton Volume

The actual volume of the skeleton of Perucetus colossus remains uncertain due to missing data. Nonetheless, Bianucci et al.’s (2023) approach, using Cynthiacetus peruvianus as a reference, is reasonable with the current available data. For instance, the mean lumbar vertebral volume of C. peruvianus is 3.29 liters (calculated from Bianucci et al., 2023, supplementary data 4), constituting 2.74% of the total vertebral column volume. On the other hand, the mean lumbar vertebral volume of P. colossus, based on the four most complete lumbar vertebrae, is 72 liters (calculated from Bianucci et al., 2023, supplementary data 4). Assuming a similar ratio between C. peruvianus and P. colossus, therefore, the vertebral column volume would be 2,628 liters. This result aligns with the estimated values for P. colossus provided by Bianucci et al. (2023), which range from 2,270 liters in their conservative model, which assumes 12 T and 15 L to 2,720 liters in their maximum estimate, which assumes 20 T and 17 L (Bianucci et al., 2023, supplementary table 22). On the other hand, they estimate a ribcage volume that should comprise from 24 to 40 ribs that falls within the range of 543 to 1,280 liters in their two P. colossus models. Although a very rough approximation, when multiplying the 16-liter posterior rib volume, which are usually smaller compared to the middle section ribs in basilosaurids (Bianucci et al., [2023], supplementary table 1) by 24, it yields 384 liters supporting their conservative model. However, the assumption of a 37 thoraco-lumbar vertebrae configuration as in C. peruvianus, for their most liberal model, very likely far exceeds the most credible vertebral number in a pachyosteosclerotic species like P. colossus (see Uhen 2001; Gingerich, 2022). Thus, their maximum estimate far surpasses the potential skeletal volume of P. colossus. Moreover, the upper estimate (1,280 liters), where the ribcage accounts for nearly half of the vertebral volume, may be excessive. Unlike P. colossus, the extant Dugong dugon, which lacks pachyostosis in its skeleton (Buffrénil et al., 2010), still exhibits notably massive ribs in comparison to its vertebral column (Figure 1I). Consequently, the ratio of rib volume to vertebral column volume is likely higher in extant sirenians than in basilosaurids, particularly with respect to pachyostotic species. According to Bianucci et al. (2023), in the skeleton SMNS-Z-MAM-1288 of D. dugon, ribs account for approximately 52% of the vertebral column volume (calculated from Bianucci et al., 2023, supplementary table 11). So, applying a similar percentage to P. colossus appears excessive. Despite that, with the current data on hand, the conservative estimation can give a good idea of the approximate skeletal volume for P. colossus; however, any result should be taken with caution.

Using Extant Whales’ Dry Skeletal Mass as a Proxy to Estimate Perucetus Mass

The use of derived whale skeletons to estimate the body mass of Perucetus colossus is inherently highly problematic. The morphometrics and specializations of living whales and P. colossus are widely distinct. Living whales’ skeletons are extraordinarily porous (Tsukrov et al., 2010; Wysokowski et al., 2020) contrary to the highly specialized P. colossus which exhibits extraordinary levels of pachyosteosclerosis in the skeleton (Bianucci et al., 2023). Consequently, the loss of water and fat from fresh skeletons to dry skeletons would have been remarkably greater in living whales compared to P. colossus, in which former wet skeletal mass makes around the 17% body mass (Nishiwaki, 1950; Lockyer, 1976). The only extant aquatic mammals known to possess highly pachyosteosclerotic skeletons are sirenians (Houssaye, 2009). Hence, using sirenians as a proxy for body mass estimation would likely yield much more reliable results than relying on extant whales. It is correspondingly advisable to avoid using derived whales’ SFs as proxies. Additionally, Bianucci et al. (2023) pointed out that SF increases with positive allometry in sirenians. However, this factor was not considered in the estimation for P. colossus. Nor did they extrapolate data from the giant recently extinct sirenian, Hydrodamalis, which exhibits an SF of about 10% (Bianucci et al., 2023: supplementary data 2). Had these considerations been incorporated, it would have markedly reduced the estimated body mass for P. colossus.

Dry Done Tissue Moisture

Bianucci et al. (2023) assumed that the dry skeletons only lack free water, which represents just the 20% of the total water in bone tissue (Surowiec et al., 2022). However, dry skeletons lose much of their water content, if not practically all (Appendix 1). In any case, Bianucci et al. (2023) did not account for water loss in their calculations of Perucetus colossus dry skeletal mass, resulting in an overestimation of the actual skeletal mass (Appendix 1).

MATERIAL AND METHODS

Volumetric Modeling

In order to test the method introduced herein, and to mass estimate Perucetus colossus, six living marine mammal taxa representing four adult cetaceans and two adult sirenians were volumetrically restored (Table 1, Figure 1, Figure 2). Aside from P. colossus, three other extinct sea mammals were also restored, including two basilosaurids: the late Eocene Basilosaurus cetoides and Cynthiacetus peruvianus; as well as the recently extinct Steller’s sea cow, Hydrodamalis gigas (Table 1, Figure 1, Figure 2). We have used both whales and sea cows, extinct and extant, because while a whale, P. colossus appears to exhibit some attributes of sirenians (Bianucci et al., 2023; Motani and Pyenson, 2024).

To achieve optimal results, the best-preserved specimens, ranging from partially complete to nearly intact skeletons, were selected. (Table 1) The lateral, dorsal, and ribcage cross-section profile-skeletals were generated using publicly available photographs and illustrations of skeletal mounts captured from lateral and dorsal perspectives. Additionally, publicly accessible 3D scans of the skeletons were employed (accessed on Sketchfab.com and Rigsters.com). When a particular specimen was nearly complete but missing certain skeletal parts, or when these parts were not sufficiently well-depicted, they were reconstructed by referencing similarly sized specimens of the same species in which those parts were preserved. Each bone was reconstructed individually, adding flesh applying comparative anatomy of extant whales and sirenians. Marine mammals are typically ensheathed in substantial deposits of blubber, and the peripheral adipose tissues and correspondingly smoothed superficial topographies were aided by publicly available lateral and aerial dorsal view photographs (as per Christiansen et al., 2020) of wild living individuals that place tight constraints on body dimensions, as well as CT scans and transverse sections of specimens (Computerized Scanning and Imaging Facility, accessed on https://csi.whoi.edu; Huggenberger et al., [2019]; Ivančić et al., [2014]). Motani and Pyenson (2024) did not use sirenians for comparative purposes. We do so because Bianucci et al. (2023) cite the significant possibility that P. colossus converged with sirenians in some regards. Taxa are profiled as being in prime condition, well-nourished and non-pregnant or non-lactating, in the case of females (see Christiansen et al., 2020). Marine mammals including cetaceans are known for having large intervertebral cartilages. In a rare examination, Flower (1868) noted that the intact vertebral column, with cartilage, in a mature porpoise was 15% longer than when the intervertebral cartilage is removed. Therefore, a factor of 1.15 was applied to determine the mean intervertebral spaces in the profile-skeletal restoration presented herein.

Because MNHN.F.PRU10 is exceptionally well-preserved and described in detail with multiview images of all the postcranial elements (Martínez-Cáceres et al., 2017), the form of C. peruvianus can be restored with a high degree of confidence. B. cetoides was restored with 15 (T), 15 (L), and 21 (Ca) vertebrae (Kellogg, 1936). The number of thoracic vertebrae appears to be correct based on the USNM 4675 skeleton, which preserves cervical and thoracic series plus the first lumbar vertebrae (Kellogg, 1936). There may be some uncertainty regarding the total number of lumbar vertebrae, but the proposed number could be correct (see Kellogg, 1936, p. 47). This basilosaurid was not restored in top view because of a surprising lack of imagery of dorsal views of elements (including in Kellogg, 1936), the resulting mass estimate is correspondingly approximate.

The skull of P. colossus is unknown, however, Gingerich et al. (2022) found an allometric correlation between the lumbar centrum length and skull length in basilosaurids. They determined that the natural logarithm (ln) of lumbar centrum length equals the ln of skull length multiplied by 1.59 minus 5.39 in basilosaurines and pachycetines. The average lumbar length in P. colossus is 333.8 mm (Appendix 2), suggesting a skull length of about 1,150 mm. If one applies the equation for dorudontines (Y = 1.93X - 8.78; where Y is the ln of skull length and X is the ln of lumbar centrum length), it results in a skull length of about 1,900 mm. Since P. colossus exhibits elongated lumbars somewhere between pachycetines and dorudontines (see Uhen 2001; Gingerich, 2022; Bianucci et al., 2023), an intermediate skull length of 1,500 mm was assumed for the profile-skeletal and body length calculations [Figure 2; Appendix 2].). Because cervical vertebrae size is closely correlated with skull length (see Appendix 2), the cervical series length for Perucetus colossus was inferred by comparing the estimated skull length (1,500 mm) with other basilosaurids (Appendix 2).

To estimate the total length of P. colossus, its most plausible vertebral configuration was determined based on pachycetines (Gingerich et al., 2022). This clade exhibits many characteristics shared by P. colossus including pachyosteosclerotic vertebrae and ribs, elongated vertebral centra, and lumbars having transverse processes nearly as long anteroposteriorly as the centra from which they arise (Gingerich et al., 2022). Therefore, P. colossus might belong to this clade, and if not, it would be very closely related or proportionally convergent. Pachycetinae with an arguably complete thoracic column have 12 to 13 thoracic vertebrae (Uhen, 2001, Gingerich et al., 2022), and thus, the same was assumed for P. colossus (Appendix 2). In basilosaurids, the number of lumbar vertebrae ranges from slightly fewer than thoracic, matching the number of thoracic vertebrae, to at most three more lumbar vertebrae than thoracic vertebrae (see Uhen, 2004; Martínez-Cáceres et al., 2017). Nevertheless, the lowest reported number of lumbar vertebrae in basilosaurids is 12 (Martínez-Cáceres et al., 2017). Therefore, two possibilities were taken into account for estimating the most plausible length variation in P. colossus: ranging from 12 T and 12 L to 13 T and 16 L vertebrae (Appendix 2). We also explored the less probable configuration present in C. peruvianus for P. colossus, with 20 T and 17 L, for comparison purposes.

As for caudals, basilosaurids typically have 21 caudal vertebrae, a number also observed in basal cetaceans like protocetids (Kellogg, 1936; Uhen, 2004; Gingerich et al., 2009). To infer the missing vertebrae centra approximate lengths of P. colossus, we used Pachyecetus wardii, Antaecetus aithai and Basilosaurus cetoides as a reference (Appendix 2).

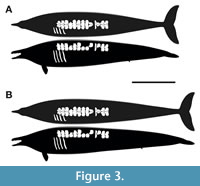As was done with the length calculations (Appendix 2), the P. colossus profile-skeletals were restored with the approximate thoracic proportions of pachycetines (Figure 1A, B, Figure 2B, and Figure 3), where the complete thoracic series of Pa. wardii is about 4 times longer than the last two trunk vertebrae (calculated from Appendix 2); the ratio appears to be also very similar in the pachycetine A. aithai, even though it has one fewer thoracic vertebra (Appendix 2) (note that the trunk centra shorten dramatically progressing anteriorly in basilosaurids). Therefore, the inferred thoracic region length, based on either Pa. wardii or A. athai, results in nearly identical measurements for P. colossus (Appendix 2). The lumbar region was based on the more complete B. cetoides, in which most of the lumbar vertebrae lengths remain relatively constant as in P. colossus and pachycetines (Appendix 2). The main profile-skeletal (Figure 1A, B, Figure 2B) tail was restored to the average length between our conservative and most liberal estimates inferred for the lumbo-caudal vertebral region (calculated from Appendix 2). Another two profile-skeletals of P. colossus were created to match the proportions of the minimum and maximum estimations (Appendix 2; Figure 3).

The soft tissue of P. colossus was restored considering two possibilities. The first one is based on living cetaceans. Given its pachyosteosclerotic skeleton, an alternative hypothesis is that the surrounding soft tissue was like that of sirenians, similarly thick to the exceptionally thick, soft tissue of polar balaenids. Hence, the second option was modeled based on the characteristics of modern, thick-skinned, warm-water sea cows.

Total lengths of profile-skeletals are measured along the body midline. These are most reliable for extant taxa, but even then, there are uncertainties of skeletal (including intervertebral spaces) to skeleton plus soft tissue lengths of a percent or two, most of all with the sperm whale because of variations in the dimensions of the immense sonar body relative to the skull.

The volumes of the skeletal profiles were measured by water displacement (WD) and graphic double integration (GDI). For the former, the skeletal reconstructions were modeled at 300 mm, a large model size suitable for accurate volume modeling. A clear plexiglass sheet was placed over each lateral profile, and a plasticine half-model was sculpted on top to match the profile precisely. The completed half-model was then sectioned and immersed in a graduated cylinder (1,000 ml) to measure its volume, with each measurement repeated to ensure accuracy. Alternatively, the volumes of the profile skeletals were estimated using (GDI), a volumetric method developed by Jerison (1969) for estimating endocast volumes from dorsal and lateral views. Jerison found that, for his brain endocast data set, GDI calculated volumes within 5% accuracy compared to the WD method. Later, other authors applied it to whole animals (Hurlburt, 1999; Larramendi, 2016). The GDI method requires two views of the model, typically the lateral and dorsal views. The model Is divided into multiple slices, with each slice’s volume calculated as an elliptical cylinder using the equation: V= π(r1)(r2)(L) where V is volume, r represents radii, and L is length. The volumes of all slices are then summed to obtain the total volume of the model.

The volume of the cetacean profile-skeletals was measured sans fins, and afterward, the volume of the tail flukes, flippers, and fins was added as an additional 1.5% in accord with direct measurements, where these vary from about 1% to 2% of the total body mass in whales (Ohno and Fujino, 1952). Because the flukes and arms of sirenians are substantially larger, a 4% estimate was assumed based on educated judgment in this case. All model volumes were calculated at the same length of 100 units, and a specific gravity of 1.025 was utilized to arrive at the masses because this density is required to approximate neutral buoyancy in marine mammals (Larramendi et al., 2021).

Each model result represents a k value, which equals the model mass in kg at a length of 100 cm. k values make it easy to compare the relative mass/length proportions of differing specimens and taxa. If one k value is twice as high as another, then the former is twice as heavy for a given length. To calculate the body mass of a specific specimen in kilograms, multiply the k value by the length in meters cubed.

Because the volumetric restorations produced here mostly represent adult individuals close to the average size, extrapolations of k values to different-sized individuals should yield the most reliable results if they are mature and close to the average size, as significant allometric trajectories or ontogenetic differences would be minimized. Therefore, extrapolations to ontogenetically significantly distinct individuals and/or far from the current restored size range, would result in less reliable outcomes.

To assess the accuracy of the obtained body mass results in the volumetrically restored extant taxa, they were compared to the body masses of individuals that had been directly weighed (Tomilin, 1947; Nishiwaki, 1950; Ohno and Fujino, 1952; Gambell, 1970; Domning and Buffrénil, 1991; Sarko et al., 2010). In the case of cetaceans, most of the reported masses do not account for fluid loss -particularly blood- post-flensing. This loss amounts to approximately 8% of the total body mass in baleen whales and at least 10% in sperm whales (Wood, 1982; Lockyer, 1976). As a result, a correction factor was applied to carcass body masses as needed.

RESULTS AND DISCUSSION

The obtained mass results for the analyzed aquatic mammals using WD and GDI methods are very similar (Table 2), with differences between the two methods ranging from 0.3 to 4.8%, favoring slightly higher results with the WD method. The updated skeletal masses for Perucetus colossus are listed in Table 3.

Extant Marine Mammal Body Masses

Starting with extant marine mammals, the actual form and proportions of Balaenoptera musculus are shown in Figure 1D and Figure 2D. In most museum skeletal mounts, the ribs have been mounted too vertically transversally and fore-and-aft in skeletal mounts, making the chest deeper and less broad when photographs show the opposite is correct, as is true of rorquals in general. Our result is a streamlined hydrodynamic form sporting a total length to maximum depth ratio of ~8 in B. musculus. The LML GOGA 1982 skeleton with a skeletal length of 26.5 m, implies a living length of about 27 m. At this length, based on the mean k value, a mass of 150.4 tonnes is computed (Table 2). According to Nishiwaki (1950) a 27-m-long blue whale (N.No.319.B.F) weighed 127.5 tonnes without blood, including it -which equals ~8% of total mass-, the weight would rise to 138.6 tonnes, roughly 8% less than the estimated mass here. In the case of another specimen with a length of 27.1 m, it weighed 136.4 tonnes (148.3 tonnes including blood) (Tomilin, 1947), closely matching the calculated result presented here for a blue whale of the same length.

The fin whale (Balaenoptera physalus) is generally similar in relative proportions to the blue whale (Figure 1E, Figure 2E), with a mass at a given length and k value being about a tenth less. Overly vertical mounting of ribs is also the norm in fin whale mounts, although the fin whale’s ribcage depth/breadth ratio is lower than that of blue whales partly because of taller dorsal neural spines. The fin whale has a relatively deeper tail than the blue whale despite having lower caudal neural spines than the latter, and the head is relatively a little smaller in B. physalus. Nishiwaki (1950) reported an average body length of 21 m and a weight of 56.2 tonnes (including blood) for the species. Our volumetric model predicts a mean weight of 61.5 tonnes at this length, which is approximately 9% higher than the observed value. However, another specimen (H.No.795. F.F.) from the same study, measured 21.3 m in length and weighed 65.5 tonnes (including blood), over 1 tonne more than predicted by our mean k value. The sample of five fin whales in Ohno and Fujino (1952) averages only a few percent less relative to length than estimated herein, with the largest individual having a higher k value.

The sperm whale sonar complex projects far anterior to the fore tip of the skull, considerably extending the overall length relative to the combined cranium and postcrania (Figure 1G, Figure 2G). Physeter macrocephalus has higher length/depth length ratios, at least 5.8, along almost their entire length than the much more streamlined rorquals, so much so that the depth of the tissues dorsal to the tops of the neural spines, and below the aft tail, appears to be considerable in sperm whales, the k value approaching twice as high as rorquals. As for its body size relationships, the average dimensions of 16 adult individuals, accounting for fluid loss, was 15.1 m in length and 42.1 tonnes in mass (calculated from Ohno and Fujino, 1952). At this length, our mean k value estimates a body mass of 45.2 tonnes, approximately 7% higher than the observed value, but some large individuals were close to and sometimes exceeded the modeled estimate. Another specimen measuring 13.3 m in length was weighed in its entirety at 31.5 tonnes (Gambell, 1970), only over 1.5% more than predicted by our k value.

Also featuring the enormous heads that distinguish all living giant whales, right whales (Eubalaena spp.) exhibit an extremely portly body form (Figure 1F, Figure 2F), the total length/depth ratio being just ~4.5 with only the aft tail being narrow. The low ratio results from the enormous depth and width of the high-capacity ribcage compared to other whales. The ribcage depth is about twice as great at a given length compared to rorquals, and the trunk cross-section is roughly subcircular, with the width being slightly less than the depth. The k value is three times that of comparatively trim rorquals, making balaenids extraordinarily heavy for their length. As a matter of fact, the only whale, aside from the blue whale, to have exceeded 100 tonnes with a piecemeal weight is the North Pacific right whale, Eubalaena japonica. A particularly massive specimen measuring 17.4 m in length weighed 106.5 tonnes (Klumov, 1962; Lockyer, 1976). The reconstructed body mass, including blood, would be around ~116 tonnes, however. At that length, the mean k value for the North Atlantic right whale, E. glacialis, a species with a very similar shape, yields a slightly lower result at 108 tonnes. However, this enormous specimen was a lactating female, which explains the high body mass for its length (see Klumov, 1962). Conversely, according to Omura et al. (1969), the mean length of 11 adult individuals of E. japonica was 15.4 m, with a reconstructed body mass of 64.2 tonnes. At this length, our mean k value suggests a 17% higher body mass. Notably, one of the specimens (63C) measuring 16.4 m in length weighed 85.3 tonnes (including blood), only 6% below what our mean k value predicts.

Sirenians are always rotund but not consistently so. The lateral and dorsal view photographs of extant and recent sirenians and their skeletons show that they have low length/depth values of between 4.5 and 5 because they have long chest ribs like those of right whales, their ratio relative to total body length being ~8 (Figure 1H-J, Figure 2H-J) and the ribs being ~60 percent longer than those of rorquals at a given length. The Florida manatee trunk is somewhat broader than deep, the ratio being ~1.25 (Figure 2I), a feature that is unusual among marine mammals. The more whale-like dugong is about as deep as it is broad (Figure 2H). Sirenian k values are in the right whale zone. Based on data from Sarko et al. (2010), the average size of six adult dugongs, Dugong dugon, is 2.51 m in length and 300 kg in mass. According to our dugong model, at this length the predicted body mass is 330 kg. However, one of the specimens (MM 89), measuring 2.65 m in length, weighed 413 kg, which is 6.4% heavier than the estimated mass by mean k value in this study (Table 2). Utilizing the data from Sarko et al. (2010), the average length for adult Florida manatees, Trichechus manatus, is 3.25 m, with a mass of 600 kg. This closely aligns with the value of 631 kg obtained using Domning and Buffrénil’s (1991) proposed equation. However, our volumetric model estimates a mass of 793 kg at this length, roughly 25% more than the expected mass. Notably, certain specimens can be exceptionally robust. For instance, UF 24969, with a length of 3.27 m, weighed 975 kg, 20% more than the 808 kg predicted using our mean k value (Table 2). Nevertheless, many of the reported lengths of manatees in Domning and Buffrénil’s (1991) and Sarko et al. (2010) were taken from data sheets filled out by Fish and Wildlife Service personnel, and those may have been along a curved-line (Daryl Domning personal commun., 2023), instead of along the body midline as our k values were derived. In our T. manatus model, the curved-line length is 7% greater than the midline length, which implies that our results would have been about 20% lower if the k value was based on body curvature. In the case of a 3.25 m T. manatus, it would have weighed 647 kg, just barely above the expected masses following Sarko et al. (2010) and Domning and Buffrénil’s (1991).

Because the results obtained through the volumetric models are usually somewhat greater than expected, this indicates that the soft tissue surrounding the skeletons is by no means underestimated, and therefore, they can be considered representing well-nourished individuals. Thus, the body mass results obtained for extinct marine mammals are unlikely to be undercalculated. At nearly identical to at most 25% difference, the variations between observed and volumetric masses are modest at worst.

Size of World-Record Blue Whales

Recently, Motani and Pyenson (2024) suggested that the largest ever recorded blue whale approached a mass of 270 tonnes. This estimate was derived from regression-based and volumetric approaches, based on the longest whale listed in Risting (1928) from the Antarctic population. It should be noted that Antarctic blue whales (B. m. intermedia) are recognized as the largest subspecies (McClain et al., 2015). The specimen in question measured 33.27 m in a straight line from the snout to the end of the tail between the tail fins. However, this specimen was captured in the South Shetlands, where most of the whales were caught by floating factories (Risting 1928). As noted by Risting (1928) and Branch et al. (2007), measurements taken on floating factories are considerably less reliable than those taken from fixed stations, as whales are processed alongside the vessel in the water. Furthermore, McClain et al. (2015) observed that due to this and other reasons, early length measurements exceeding 30.5 m should be viewed with skepticism. Scheffer, (1974) also questioned the extreme lengths listed in Risting (1928). It is very interesting to note that when fleets went out with biologists, blue whales over 30.5 m in length all disappeared from records (Trevor Branch, personal commun., 2024; see also Mackintosh and Wheeler 1929; Nishiwaki, 1950; Ohno and Fujino, 1952), even though biologists were likely on board only on rare occasions. On the other hand, in mammals, world-record-sized individuals appear to grow up to about 25% taller or longer than the average of a given adult population, and these individuals are exceptionally rare, perhaps occurring only among hundreds of thousands or even millions (Paul, 1997; Larramendi, 2016). The mean body length for Antarctic blue whale females is around 26 m (Branch et al., 2007), suggesting that a world-record specimen might reach up to 33 m, as observed in Risting’s (1928) specimen. However, because the current or even past Antarctic blue whale populations were appreciably smaller than the aforementioned numbers (Branch et al. 2004), the probability of a blue whale of this size class occurring is very unlikely. For all these reasons, the reported measurement of 33 m is not credible for the maximum size of the blue whale. Therefore, it is advisable not to use lengths exceeding 30.5 m for mass estimation given the current data available. At this length, our k value suggests a blue whale approaching 217 tonnes. However, it must be noted that our k value for the blue whale is on the higher end for the species, and furthermore, exceptionally large individuals do not grow isometrically, but with strong allometry, being proportionally markedly lighter, at least in terrestrial animals (Larramendi, 2016). This might also hold true for whales significantly above average. For example, one of the largest blue whales ever recorded measured 29.5 m in length and had a reconstructed body mass, including fluid loss, of 178 tonnes (Scheffer, 1974), which is 10% lower than predicted by our k value. Therefore, if allometry applies to exceptionally large individuals, a blue whale at 30.5 m would be close to 200 tonnes but somewhat below at around 195 tonnes. Nevertheless, the heaviest recorded weight for a blue whale is 190 tonnes in an individual “only” 27.6 m in length (Voronin, 1948; Lockyer, 1976). However, this individual was pregnant and exceptionally fat indicating that world-record specimens, when pregnant, can exceed 200 tonnes in body mass, though likely not by a substantial margin.

We would like to emphasize that focusing on outlier individuals can create the false impression that they are representative of the species among the general public or even researchers. However, they are not; these are extremely rare cases in nature and should be treated and noted as such. Additionally, unless a fossil species is so abundant that its average body size can be estimated, and from there, estimate the limit size for the species, it is potentially misleading to compare extant world-record specimens with fossil specimens. This is because the latter will at best hardly ever preserve individuals of this unusual size category.

Perucetus colossus and Other Basilosaurids Body Size

More closely related to Paleogene Perucetus colossus than living and recent marine mammals are the serpentine bodied Basilosaurus cetoides, and the less gracile basilosaur Cynthiacetus peruvianus (Figure 1C, Figure 2C). The latter’s well-preserved and readily articulated ribcage with its strongly arced, short ribs was slightly oval, the breadth being a little less than depth. The short, well arced ribs of P. colossus indicate it had a similarly modest volume subcircular ribcage.

The C. peruvianus profile-skeletal restoration exhibits a body length of 8.95 m (Figure 2), including intervertebral cartilage (Appendix 2). In this case, the anatomical length corresponds to about 98% of the calculated living length in a straight line (Appendix 2). As expected for its elongated body plane, the k value at 5.7 is significantly lower than that of any reported extant whale (Table 2), implying a body mass of 4 tonnes for the MNHN.F.PRU10 individual.

The total length of Basilosaurus cetoides USNM 4674/4675/12261 profile-skeletal is 18.35 m (Figure 2), indicating again that the anatomical length is about 2% shorter than the expected straight living length (Appendix 2). Since it has likely a greater number of vertebrae that are, on average, about 20% longer than those of P. colossus (Appendix 2), P. colossus cannot have exceeded the length of B. cetoides, and should have been substantially shorter. On the other hand because the body length of B.cetoide USNM 4674/4675/12261 is about twice that of C. peruvianus and because the rib lengths and vertebral transverse diameters are about a third larger (see Kellogg, 1936; Martínez-Cáceres et al., 2017), the k value of B. cetoides would have been around 2.5, suggesting a body mass of approximately 15 tonnes.

The estimated living body length of Perucetus colossus ranges from 15 to 16.4 m, with a mean of 15.7 m (Appendix 2). The minimum estimate corresponds to 12 thoracic and 12 lumbar vertebrae, while the upper limit assumes 13 thoracic and 16 lumbar vertebrae. The correlation between a high number of lumbar vertebrae and increased swimming speed in modern cetaceans (Martínez-Cáceres et al., 2017) suggests that the more liberal estimate may be less applicable to the large, slow-swimming P. colossus, which likely inhabited a coastal environment (see Bianucci et al., 2023). In contrast, based on gracile dorudontines like C. peruvianus, with low bone density and very high number of thoraco-lumbar vertebrae, resulted in an estimated length of 20 m (Appendix 2), on which Bianucci et al. (2023) based their higher-end estimate. However, as pointed above, P. colossus, analogous to pachycetines with fewer trunk vertebrae, likely had a significantly shorter trunk, making an elongated 20 m body length very unlikely.

The main profile skeletal reconstruction, following the procedures outlined in the Materials and Methods, results in an animal measuring 15.7 m (Figure 1A, B, and Figure 2B). The sum of the inferred skull, cervical, and thoracic series, plus flesh and intervertebral spaces, is just over 5,000 mm assuming a straight line (calculated from Appendix 2). Restoring the tail to the average length between our conservative and most liberal estimates for the lumbo-caudal vertebral region (calculated from Appendix 2) gives a total straightline length of nearly 10,600 mm, including intervertebral spaces (this is the same approximate ratio between the preserved vertebrae and restored tail seen in the Bianucci et al. [2023, their figure 1a] skeletal). Assuming about 400 mm of flesh just behind the last caudal vertebra as normal for flukes at this body size results in a total straightline length of ~16 m which translates to around 15.7 m in anatomical posture. This is within the most plausible length range as discussed above and coincides with the calculated mean length. Therefore, our estimates indicate that Perucetus colossus was only about half the length of the longest blue whales, and could have been only a small fraction of the latter’s mass.

Despite only the posterior ribs being preserved in the P. colossus holotype skeleton, they provided sufficient information for volumetric restoration. First of all, in marine mammals, all ribs’ distal ends converge towards the bottom, closing distally, which allows placing the ribs in a reasonably correct position (Figure 1, Figure 2). Therefore, widening the body plane significantly beyond what is restored here would not be justified. On the other hand, in sirenians and cetaceans, including the species closely related to P. colossus, C. peruvianus, the body breadth remains relatively constant all around the ribcage (Figure 1, Figure 2). Therefore, the currently available data offers adequate information to approximate the volume of P. colossus.

The estimated mean body mass for P. colossus at a length of 15.7 m is approximately 36.7 tonnes in the cetacean-like soft tissue option and 40.5 tonnes in the sirenian-like soft tissue alternative (Figure 2B). When modifying the main profile-skeletal to match the minimum length estimation (15 m) (see Figure 3B; Appendix 2), the body mass estimation drops to 35.3 tonnes in the cetacean-like soft tissue model and 38.8 tonnes in the sirenian-like soft tissue restoration. On the other hand, increasing the profile-skeletal proportion to match the maximum length estimation (16.4 m), the body above masses increase to 38.5 and 42.2 tonnes in the two types of soft tissue restorations. It is noteworthy, that since our volumes/masses are already at a high level in the rest of the restorations, is not viable that P. colossus would be much higher than calculated here in non-pregnant condition. With the caveat that P. colossus and other basilosaurids probably had thinner fat deposits than modern whales, as they swam in the much warmer seas of the Paleocene. In such a warm environment, very thick blubber could have been counterproductive for large cetaceans, especially basal forms, due to the increasing difficulty of dissipating heat caused by the scaling relationship between body surface area and volume (Schmidt-Nielsen, 1984). Sea cows have unusually low metabolic rates for mammals (Noren and Rosen, 2023), leaving them dangerously vulnerable to hypothermia even in middle latitude winters (Bossart et al., 2002). It is presumed in this study that basilosaur energetics were in the higher cetacean range, in which case fat deposits should not have been heavy and body masses may have been yet lower than the minimum presented here. So, 35 tonnes or below for P. colossus cannot be ruled out. Conversely, masses above 50 tonnes than found here would be difficult to defend based on the length and, particularly, thickness of Perucetus colossus. For instance, even if we were to double the thickness of the surrounding soft tissue in our most liberal restoration, which is inherently unreliable, the resulting body mass would still be 58 tonnes.

Furthermore, the revised dry skeletal mass of P. colossus falls within the range of 4.3 to 6.15 tonnes (Table 3), which is more than a fifth less than the estimate provided by Bianucci et al. (2023). However, the upper limit, based on an improbable number of vertebrae for P. colossus, is highly implausible and should not be utilized. Since the lower estimate assumes 15 lumbar vertebrae, it may overestimate the dry skeletal mass of P. colossus, as the animal may have had only 12 lumbar vertebrae. Given that the average lumbar volume in P. colossus is 72 liters (calculated from Bianucci et al., 2023, supplementary data 4), excluding three lumbar vertebrae would reduce the vertebral volume to 2,054 liters, it results in a dry skeletal mass of 4.0 tonnes. The lower end skeletal dry masses align with our estimated body mass, in which the SF in other giant pachyosteosclerotic clades such as Hydrodamalis gigas, comprises 9% of the body mass assuming the estimated skeletal mass is reasonable (Appendix 1). Applying this proportion to P. colossus, a body mass ranging from 44 to 48 tonnes is obtained. An even significantly more conservative estimate would be reached if positive allometry of SF, as appears to be in sirenians inter-specifically, is considered (Appendix 1). Consequently, the assignment of a blue whale size class to P. colossus is not supported by the available evidence. Even the sperm whale exhibits a k value significantly higher than that of P. colossus, meaning that at the same length, the sperm whale easily outweighs P. colossus.

Despite the dry skeletal mass being proportionally much heavier in Perucetus than in Balaenoptera, this does not necessarily mean that the former must be heavier because pachyostotic species are actually proportionally lighter in body mass for their dry skeletal mass (Motani and Pyenson, 2024), as they can compensate for their high skeletal density through large lung volumes (Kipps et al., 2002). Otherwise, the k value, or the relative body mass found in Eubalaena, would have been much lower than that of sirenians but is not, nor is the surrounding soft tissue around the skeleton smaller in this cetacean compared to sirenians (Figure 1, Figure 2; see also Motani and Pyenson, 2024). Therefore, much higher estimates are not supported by the available fossil evidence.

According to our model, the Bianucci et al. (2023) restoration does not match their own minimum calculated length estimate. This is because the core of the P. colossus fossil consists of 13 vertebrae that, using the reported vertebral centra lengths and adding a calculated mean intervertebral spacing of 34 mm (Appendix 2), measures approximately 4.7 m. Using this length for scaling, the profile-skeletal provided by Bianucci et al. (2023) is only ~16.5 m long, despite being drawn with a long, basilosaurine-style trunk. At this length, the schematic restoration by Bianucci et al. (2023) would weigh ~60 tonnes (Motani and Pyenson, 2024), failing to meet their minimum body mass calculation. Being based on that skeletal the Motani and Pyenson (2024) tonnages are also too high. They also err by simply isometrically scaling the skeletal in all dimensions up and down by 18%, when only the length should be varied while maintaining the same transverse dimensions of the preserved bones material and associated flesh. Moreover, the entire reconstructed thoracic trunk is about 5.5 times longer than the combined length of the two preserved posteriormost trunk centra, instead of the more likely ~4 times observed in pachycetines.

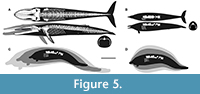
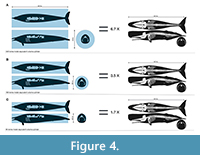To arrive at the Bianucci et al. (2023) minimum of 85 tonnes at 15.7 m requires a length/depth ratio like that of the much heftier, colossal skulled right whale. To achieve even higher masses at the lengths restored by Biancucci et al. (2023) and herein requires increasingly biologically nonviable mounds of flesh. Bianucci et al.’s (2023) 180 tonnes median value, that of a very large blue whale, demands the length/depth ratio of 3 not seen in even the stoutest actual marine mammals including the right whale, and in absolute measure is broader than the far larger blue whale. To put it differently, the 180 tonnes estimate requires a 15.7-m-long P. colossus to be as voluminous as a cylinder of the same length and with a diameter of 3.8 m (equating to 178 m3; Figure 4), or over three times more voluminous than a sperm whale of same length (Figure 4). Even the thickest part of P. colossus is much thinner than the average thickness of a cylinder of that size (Figure 4). Even for the extraordinarily massively built Trichechus, at a hypothetical 15.7 m in length, it would weight “only” 89 tonnes. The 341 tonnes maximum demands a yet more patently unrealistic length/diameter ratio of around two (Figure 5). Applying right whale/sirenian-like proportions at the 341 tonnes that exceeds the less corpulent anatomically realistic restoration by about tenfold, forces the length to be over twice that restored here (Figure 5). We present such extreme cross comparisons to demonstrate the implausibility of these anatomical scenarios, as well as to emphasize the need to produce realistic volumetric models; Motani and Pyenson (2024) made similar observations.

P. colossus, from the Bartonian stage of the Eocene, is one of the earliest known cetaceans to possess fully developed fins and a completely marine lifestyle. When such features first appeared is not certain due to geological gaps in the marine fossil record, but it was probably only ~10 million years prior (Fordyce 2018). The revised, lesser body size for P. colossus puts it more in line with that of the previously known big basilosaurids. The basal cetacean size rise from a few tens or hundreds of kilograms to tens of tonnes over such a short span is a remarkable example of the trend toward larger body sizes observed in marine animals during their evolution (Heim et al., 2015). But it is not the extraordinary expansion that it would be if the Bianucci et al. (2023) estimations were considered, so similarly exceptional productivity of Eocene oceans need not be invoked as an explanation (see Motani and Pyenson, 2024). Instead, whales did not supersize until truly atypical ice age maritime conditions did promote such at the end of the Neogene (Slater et al., 2017). A similarly unlikely rapid size expansion, likewise supposedly stimulated by unusually high marine productivity in the earliest Mesozoic, has been presented (Sander et al., 2021; Lomax et al., 2018) and contested with similar profile-skeletals of the earliest Mesozoic marine reptiles (Paul 2022) that found none of which to have exceeded 20 tonnes.

CONCLUSION

According to our findings, utilizing comparative anatomy and the presently accessible data, the volumetric restoration of the Perucetus colossus MUSM 3248 fossil indicates a body mass in the range of 35 to 40 tonnes. This estimate is further supported by the reviewed skeletal mass of the type skeleton and the SF found in large pachyosteosclerotic marine taxa. While there is no doubt that P. colossus individuals may have exceeded the size of the holotype, our estimated size range is far lower than 85 to 340 tonnes estimated by Bianucci et al. (2023). It is also below the 60 to 70 tonnes favored by Motani and Pyenson (2024), who also suggested a more realistic ~40 tonnes. In order to propose estimates well above 40 tonnes for MUSM 3248, it is needed to present biologically plausible profile-skeletals that convincingly illustrate such. The method employed by Bianucci et al. (2023) to try to estimate the mass of P. colossus raised doubt because the results varied by a factor of four, a divergence not found among other palaeontological methods. As it is, using the skeletal/total mass ratios observed in sirenians with high density bones that are broadly similar to those of P. colossus produces mass values fairly close to those derived from volumetric methods with higher likelihood. Therefore, this approach may produce more likely results when comparing with a sufficiently consistent bone density value range in the fossil vis-à-vis modern analogs.

It is unambiguous that there is no current evidence that P. colossus came close to approaching the size of average blue whales of 100 to 150 tonnes. According to our estimates, the size of the Paleogene Peruvian whale holotype was fairly normal for Eocene to Miocene whales and is in the range of sei, gray, and humpback whales (Ellis, 1980; McClain et al., 2015). The blue whale easily remains unchallenged as the largest known marine animal. As for the largest animal of all, that is uncertain because fragmentary remains suggest that some sauropod dinosaurs matched the mass of blue whales, their immense size supported by a stable diet of tall tree forests (Paul and Larramendi, 2023).

High-end claims of extraordinary sizes based on partial fossil remains should be accompanied with specimen-specific profile-skeletal restorations that demonstrate that the particular high values are anatomically reasonable for the specific specimen/s of concern.

We hope that the technical marine mammal profile-skeletals provided here can be used to aid size estimations of future finds. They can also be used by marine artists to more accurately and consistently illustrate the marine titans of modern oceans.

ACKNOWLEDGEMENTS

Thanks to M. Schweitzer and D. Wescott for their insights into moisture levels and shrinking in dry and fossil bones, and to D. Domning for clarifying uncertainties in the measurements of Florida manatees. Thanks also go to T. Branch and L. Graham III for their insights on the largest blue whales and for sourcing literature. Finally, we thank the anonymous reviewers and Associate Editor G. Sobral for their critical review of the manuscript, which significantly improved this study.

REFERENCES

Bianucci, G., Lambert, O., Urbina, M., Merella, M., Collareta, A., Bennion, R., Salas-Gismondi, R., Benites-Palomino, A., Post, K., de Muizon, C., Bosio, G., Di Celma, C., Malinverno, E., Pierantoni, PP., Villa, I. M., and Amson, E. 2023. A heavyweight early whale pushes the boundaries of vertebrate morphology. Nature, 620(7975):824-829.
https://doi.org/10.1038/s41586-023-06381-1

Bigg, M.A. and Wolman, A.A. 1975. Live-capture killer whale (Orcinus orca) fishery, British Columbia and Washington, 1962-73. Journal of the Fisheries Board of Canada, 32(7):1213-1221.
https://doi.org/10.1139/f75-140

Bossart, G.D., Meisner, R.A., Rommel, S.A., Ghim, S., and Jenson, A.B. 2002. Pathological features of the Florida manatee cold stress syndrome. Aquatic Mammals, 29:9-17.
https://doi.org/10.1578/016754203101024031

Branch, T.A., Matsuoka, K., and Miyashita, T. 2004. Evidence for increases in Antarctic blue whales based on Bayesian modelling. Marine Mammal Science, 20(4):726-754.
https://doi.org/10.1111/j.1748-7692.2004.tb01190.x

Branch, T.A., Abubaker, E.M.N., Mkango, S., and Butterworth, D.S. 2007. Separating southern blue whale subspecies based on length frequencies of sexually mature females. Marine Mammal Science, 23(4):803-833.
https://doi.org/10.1111/j.1748-7692.2007.00137.x

Brassey, C.A. 2017. Body-mass estimation in paleontology: a review of volumetric techniques. The Paleontological Society Papers, 22:133-156.
https://doi.org/10.1017/scs.2017.12

Cambell, R. 1970. Weight of a sperm whale, whole and in parts. South African Journal of Science, 66(7):225-227.

Christiansen, F., Vivier, F., Charlton, C., Ward, R., Amerson, A., Burnell, S., and Bejder, L. 2018. Maternal body size and condition determine calf growth rates in southern right whales. Marine Ecology Progress Series, 592:267-281.
https://doi.org/10.3354/meps12522

Christiansen, F., Dawson, S.M., Durban, J.W., Fearnbach, H., Miller, C.A., Bejder, L., Uhart, M., Sironi, M., Corkeron P., Rayment, W., Leunissen, E., Harai, E., Ward, R., Warick, H.A., Kerr, I., Lynn, M.S., Pettis, H.M., and Moore, M.J. 2020. Population comparison of right whale body condition reveals poor state of the North Atlantic right whale. Marine Ecology Progress Series, 640:1-16.
https://doi.org/10.3354/meps13299

Ellis, R. 1980. The Book of Whales. Alfred A. Knopf, New York.

Flower, W.H. 1868. XII. On the Osteology of the Cachalot or Sperm-Whale (Physeter macrocephalus). The Transactions of the Zoological Society of London, 6(6):309-372.
https://doi.org/10.1111/j.1096-3642.1868.tb00580.x

Fordyce, R.E. 2018. Cetacean evolution, p. 180-185. In Würsig, B., Thewissen, J.G.M., and Kovacs, K.M. (Eds). In Encyclopedia of Marine Mammals, Academic Press, San Diego, California.
https://doi.org/10.1016/C2015-0-00820-6

Gingerich, P.D., Haq, M-u., Koenigswald, W., Sanders, W.J., Smith, B.H., and Zalmout, I.S. 2009. New protocetid whale from the middle Eocene of Pakistan: birth of land, precocial development, and sexual dimorphism. PLoS ONE, 4:e4366.
https://doi.org/10.1371/journal.pone.0004366

Gingerich, P.D., Amane, A., and Zouhri, S. 2022. Skull and partial skeleton of a new pachycetine genus from the Aridal Formation, Bartonian middle Eocene, of southwestern Morocco. PLoS ONE, 17:e0276110.
https://doi.org/10.1371/journal.pone.0276110

Heim, N.A., Knope, M.L., Schaal, E.K., Wang, S.C., and Payne, J.L. 2015. Cope’s rule in the evolution of marine animals. Science, 347(6224):867-870.
https://doi.org/10.1126/science.1260065

Houssaye, A. 2009. “Pachyostosis” in aquatic amniotes: a review. Integrative Zoology, 4(4):325-340.
https://doi.org/10.1111/j.1749-4877.2009.00146.x

Huggenberger, S., Oelschläger, H., and Cozzi, B. 2019. Regional anatomy, development, and hydrodynamics including skin anatomy, p. 5-135. In Huggenberger, S., Oelschläger, H., and Cozzi, B. (eds.), Atlas of the Anatomy of Dolphins and Whales. Academic Press.
https://doi.org/10.1016/b978-0-12-802446-1.00002-3

Hurlburt, G. 1999. Comparison of body mass estimation techniques, using recent reptiles and the pelycosaur Edaphosaurus boanerges. Journal of Vertebrate Paleontology, 19(2):338-350.
https://doi.org/10.1080/02724634.1999.10011145

Ivančić, M., Solano, M., and Smith, C.R. 2014. Computed tomography and cross-sectional anatomy of the thorax of the live bottlenose dolphin (Tursiops truncatus). The Anatomical Record, 297(5):901-915.
https://doi.org/10.1002/ar.22900

Kellogg, R. 1936. A Review of the Archaeoceti. Issue 482 of Carnegie Institution of Washington, Washington, D.C.

Kipps, E.K., Mclellan, W.A., Rommel, S.A., and Pabst, D.A. 2002. Skin density and its influence on buoyancy in the manatee (Trichechus manatus latirostris), harbor porpoise (Phocoena phocoena), and bottlenose dolphin (Tursiops truncatus). Marine Mammal Science, 18(3):765-778.
https://doi.org/10.1111/j.1748-7692.2002.tb01072.x

Klumov, S.K. 1962. The right whales in the Pacific Ocean. Biological marine studies. Trudy Institute Okeanog, 58:202-297.

Larramendi, A. 2016. Shoulder height, body mass, and shape of proboscideans. Acta Palaeontologica Polonica, 61(3):537-574. https://doi.org/10.4202/app.00136.2014
https://doi.org/10.4202/app.00136.2014

Larramendi, A., Paul, G.S., and Hsu, S.Y. 2021. A review and reappraisal of the specific gravities of present and past multicellular organisms, with an emphasis on tetrapods. The Anatomical Record, 304(9):1833-1888.
https://doi.org/10.1002/ar.24574

Lister, A.M. and Stuart, A.J. 2010. The West Runton mammoth (Mammuthus trogontherii) and its evolutionary significance. Quaternary International, 228(1-2):180-209.
https://doi.org/10.1016/j.quaint.2010.07.032

Lockyer, C. 1976. Body weights of some species of large whales. ICES Journal of Marine Science, 36(3):259-273. https://doi.org/10.1093/icesjms/36.3.259

Lockyer, C. 1981. Growth and energy budgets of large baleen whales from the Southern Hemisphere. Food and Agriculture Organization, 3:379-487.

Lomax, D.R., De la Salle, P., Massare, J.A., and Gallois, R. 2018. A giant Late Triassic ichthyosaur from the UK and a reinterpretation of the Aust Cliff ‘dinosaurian’ bones. PLoS ONE, 13(4):e0194742.
https://doi.org/10.1371/journal.pone.0194742

Mackintosh, N.A. and Wheeler, J.F.F. 1929. Southern Blue and Fin Whales. Cambridge University Press, 1:1-77.

Martínez-Cáceres, M., Lambert, O., and de Muizon, C. 2017. The anatomy and phylogenetic affinities of Cynthiacetus peruvianus, a large Dorudon-like basilosaurid (Cetacea, Mammalia) from the late Eocene of Peru. Geodiversitas, 39(1):7-163.
https://doi.org/10.5252/g2017n1a1

Mattioli, S. and Domning, D.P. 2006. An annotated list of extant skeletal material of Steller's sea cow (Hydrodamalis gigas) (Sirenia: Dugongidae) from the Commander Islands. Aquatic Mammals, 32(3):273-288.
https://doi.org/10.1578/am.32.3.2006.273

McClain, C.R., Balk, M., Benfield, M.C., Branch, T.A., Chen, C., Cosgrove, J., Dove, A.D.M., Gaskins, L., Helm, R.R., Hochberg, F.G., Lee, F.B., Marshall, A., McMurray, S.E., Schanche, C., Stone, S.N., and Thaler, A.D. 2015. Sizing ocean giants: patterns of intraspecific size variation in marine megafauna. PeerJ, 3:e715.
https://doi.org/10.7717/peerj.715

Mikhalev, Y. 2019. Analysis of the Correlation Between Whale Length and Weight. Whales of the Southern Ocean: Biology, Whaling and Perspectives of Population Recovery, 5:31-62.
https://doi.org/10.1007/978-3-030-29252-2_2

Motani, R. 2023. Paleomass for R-bracketing body volume of marine vertebrates with 3D models. PeerJ, 11:e15957. https://doi.org/10.7717/peerj.15957

Motani, R. and Pyenson, N.D. 2024. Downsizing a heavyweight: factors and methods that revise weight estimates of the giant fossil whale Perucetus colossus. PeerJ, 12:e16978.
https://doi.org/10.7717/peerj.16978

Narazaki, T., Isojunno, S., Nowacek, D.P., Swift, R., Friedlaender, A.S., Ramp, C., Smout, S., Aoki, K., Deecke, V.B., Sato, K., and Miller, P.J. 2018. Body density of humpback whales (Megaptera novaengliae) in feeding aggregations estimated from hydrodynamic gliding performance. PLoS One, 13(7):e0200287.
https://doi.org/10.1371/journal.pone.0200287

Nishiwaki, M. 1950. On the body weight of whales. Scientific Reports of the Whales Research Institute, 4:184-209.

Noren, S.R. and Rosen, D.A. 2023. What are the metabolic rates of marine mammals and what factors impact this value: A review. Conservation Physiology, 11(1):coad077.
https://doi.org/10.1093/conphys/coad077

Ohno, M. and Fujino, K. 1952. Biological investigation on the whales caught by the Japanese Antarctic whaling fleets, season 1950/51. Scientific Reports of the Whales Research Institute, Tokyo, 7:125-188.

Omura, H., Ohsumi, S., Nemoto, T., Nasu, K., and Kasuya, T. 1969. Black right whales in the North Pacific. Scientific Reports of the Whales Research Institute, 21:1-78.

Paul, G.S. 1997. Dinosaur models: the good, the bad, and using them to estimate the mass of dinosaurs, p. 129-154. In Wolberg, D.L., Stump, E., and Rosenberg, G.D. (eds.), DinoFest international proceedings. Academy of Natural Sciences Philadelphia.

Paul, G.S. 2019. Determining the largest known land animal: A critical comparison of differing methods for restoring the volume and mass of extinct animals. Annals of Carnegie Museum, 85(4):335-358.
https://doi.org/10.2992/007.085.0403

Paul, G.S. 2022. The Princeton Field Guide to Mesozoic Sea Reptiles. Princeton University Press.

Paul, G.S. and Larramendi, A. 2023. Body mass estimate of Bruhathkayosaurus and other fragmentary sauropod remains suggest the largest land animals were about as big as the greatest whales. Lethaia, 56(2):1-11.
https://doi.org/10.18261/let.56.2.5

Renne, P. R., Deino, A. L., Hilgen, F. J., Kuiper, K. F., Mark, D. F., Mitchell III, W. S., Morgan, L. E., Mundil, R., and Smit, J. 2013. Time scales of critical events around the Cretaceous-Paleogene boundary. Science, 339:694-687.
https://doi/10.1126/science.1230492

Rice, D.W. and Wolman, A.A. 1971. The life history and ecology of the gray whale (Eschrichtius robustus). American Society of Mammalogists.
https://doi.org/10.5962/bhl.title.39537

Risting, S. 1928. Whales and whale foetuses: statistics of catch and measurements collected from the Norwegian whalers’ association 1922-25. Rapports et procès-verbaux des réunions, Conseil international pour l’exploration de la mer, 50:1-122.

Romano, M., Bellucci, L., Antonelli, M., Manucci, F., and Palombo, M.R. 2023. Body mass estimate of Anancus arvernensis (croizet and Jobert 1828): comparison of the regression and volumetric methods. Journal of Quaternary Science, 38(8):1357-1381.
https://doi.org/10.1002/jqs.3549

Sander, P.M., Griebeler, E.M., Klein, N., Juarbe, J.V., Wintrich, T., Revell, L.J., and Schmitz, L. 2021. Early giant reveals faster evolution of large body size in ichthyosaurs than in cetaceans. Science, 374(6575):eabf5787.
https://doi/10.1126/science.abf5787

Sarko, D.K., Domning, D.P., Marino, L., and Reep, R.L. 2010. Estimating body size of fossil sirenians. Marine Mammal Science, 26(4):937-959.
https://doi.org/10.1111/j.1748-7692.2010.00384.x

Scheffer, V.B. 1974. The largest whale. Defenders of Wildlife International, 49:272-274.

Schmidt-Nielsen, K. 1984. Scaling: Why Is Animal Size So Important? Cambridge University Press.

Slater, G.J., Goldbogen, J.A., and Pyenson, N.D. 2017. Independent evolution of baleen whale gigantism linked to Plio-Pleistocene ocean dynamics. Proceedings of the Royal Society B: Biological Sciences, 284(1855):20170546.
https://doi.org/10.1098/rspb.2017.0546

Surowiec, R.K., Allen, M.R., and Wallace, J.M. 2022. Bone hydration: How we can evaluate it, what can it tell us, and is it an effective therapeutic target? Bone reports, 16:101161.
https://doi.org/10.1016/j.bonr.2021.101161

Tomilin, A.G. 1947. Mammals of the USSR and Adjacent Countries, Vol. IX. Moskva: Izdatel'stvo Akademi Nauk SSSR, Cetacea (Translated from Russian).

Tont, S.A., Pearcy, W.G., and Arnold, J.S. 1977. Bone structure of some marine vertebrates. Marine biology, 39:191-196.
https://doi.org/10.1007/BF00387004

Tsukrov, I., DeCew, J.C., Baldwin, K., Campbell-Malone, R., and Moore, M.J. 2009. Mechanics of the right whale mandible: full scale testing and finite element analysis. Journal of Experimental Marine Biology and Ecology, 374(2):93-103.
https://doi.org/10.1016/j.jembe.2009.03.012

Tyack, P.L. 2001. Marine mammal overview, p. 1611-1621. In Steele, J.H., Turekian, K.K., and Thorpe, S.A. (eds.), Encyclopedia of ocean sciences. Academic, San Diego, California.
https://doi.org/10.1016/b978-012374473-9.00426-4

Uhen, M.D. 2001. New Material of Eocetus wardii, from the middle Eocene of North Carolina. Southeastern Geology, 40:135-148.

Uhen, M.D. 2004. Form, function, and anatomy of Dorudon atrox: An archaeocete from the middle to late Eocene of Egypt. University of Michigan Papers of Paleontology, 34:1-222.

Wysokowski, M., Zaslansky, P., and Ehrlich, H. 2020. Macrobiomineralogy: Insights and enigmas in giant whale bones and perspectives for bioinspired materials science. ACS Biomaterials Science and Engineering, 6(10):5357-5367.
https://doi.org/10.1021/acsbiomaterials.0c00364